Schlagwörter:Differentiability CalculusDifferential of A FunctionDifferentiable Function
Differentiability
Continuity and differentiability is an important idea tested on the AP Exam! Students must be able to justify continuity and differentiability using limit definitions, identify places of discontinuity and non-differentiability from a graph, and find values that would make a function continuous or differentiable at a given x-value.First, we consider the relationship between differentiability and continuity. Who are the experts? Experts have been vetted by Chegg as specialists in this subject. In the previous section, you recognized graphically the derivative of sine.

Now that we can graph a derivative, let’s examine the behavior of the graphs.Unit 2 Differentiation: Definition and Fundamental Properties >.8 The Product RuleDifferential cost refers to the difference between the cost of two alternative decisions. 3 Select the correct answer below Prtsen Home End Knewton Coursework) Differently # t PrtScn Home de End in . Unit 5 Applying derivatives to analyze functions. Moreover, a new definition of fuzzy integral called granular integral is defined, and its relation with the granular derivative is given.3—Differentiation Rules.For free notes and practice problems, visit the Calculus course on http://www. While not true for all, .4 Differentiability and Continuity • Understand the relationship between differentiability and continuity: 6 7: AP STYLE QUIZ. f ( x 2 x 2 + 3 x − 1.The derivative f‘ (a) at a specific point x=a\text {,} being the slope of the tangent line to the curve at x=a\text {,} and.Video ansehen10:05This video discusses the concept of differentiability with a variety of graphs and examples.

File Size: 294 kb. Differentiability.What is the differentiability of ? Taken f as the same as above, can f(x, y, z) = 0 be solved for (y, ) in terms of x near (0,0,1)? That is, does there exist a function : R2R such that.OptiFine – Minecraft performance tuning and advanced graphics. When x ≠ 0, the function is given by a polynomial.Autor: rogerthemathnerd File Size: 274 kb.Get free NCERT Solutions for Mathematics Class 12 Chapter 5 Continuity and Differentiability solved by experts. f ( x 2 x 2 − 13 x − 1. The tangent line to the graph of a differentiable function . For example, previously we found that d d x ( x) = 1 2 x d d x ( x) = 1 2 x by using a process . d [ sin x ] = cos x dx.6 Derivative Rules: Constant, Sum, .Schlagwörter:DifferentiabilityDifferentiable
Calculus (Version #2)
There is a natural extension to functions of three or more variables. Final Exam Math 104: Calculus Status: Not Started. DIFFERENTIABILITY OF SOME. The derivative as a function, f‘ (x) as defined in Definition 2.AP®︎/College Calculus AB 10 units · 164 skills.
CC Differentiability
4 – Connecting Differentiability and Continuity: Determining When Derivatives Do and Do Not Exist 2. We will see that if a function is differentiable at a point, it must be continuous there; however, a function that is continuous at a point need not be differentiable at that point. • Interpret rates of change over an interval or at a single point by graphical and . Unit 1 Limits and continuity. Hence, 0 lim ( ) x f x → = 3 0 lim ( 3) 0 3 3 x x → + = + = Since the limit of f at x = 0 does not coincide wit h f(0), the function is not continuous at x = 0. Example 1 Compute the differentials for each of the following functions. Of course, if we have f‘ (x) then we can always recover the derivative at a specific point by substituting x=a\text {. File Type: pdf. Take Exam More Quizzes .2 – Defining the Derivative of a Function and Using Derivative Notation 2.LESSON & OBJECTIVES.If a and b are any 2 points in an interval on which f is differentiable, then f‘ takes on every value between f‘ (a) and f‘ (b).A few AP Multiple Choice Questions (MCQs) covering the differentiability and continuity. Show transcribed image text. 15K views 6 years ago Calculus: Unit 2 – The Derivative.Use the total differential to approximate the change in a function of two variables. For instance, given the function w = g(x,y,z) w = g ( x, y, z) the differential is given by, dw = . View the full answer. Unit 2 Differentiation: definition and basic derivative rules.5 Applying the Power Rule.Schlagwörter:The Derivative of A FunctionDerivatives and Differentiation
Calculus Test Prep
6 Product and Quotient Rules • Apply the product and .7K subscribers. First, we consider the relationship between differentiability and continuity.5 – Applying the Power Rule 2. Available here are Chapter 5 – Continuity and Differentiability Exercises Questions with Solutions and detail explanation for your practice before the examination . Chapter 6 Indeterminate Forms Ex 6.In mathematics, a differentiable function of one real variable is a function whose derivative exists at each point in its domain.Schlagwörter:Differentiable Function ExamplesFunction That Is Not Differentiable2020 Mathematics Subject Classification: 05C75.
Version #1
In this paper, using the concept of horizontal membership functions, a new definition of fuzzy derivative called granular derivative is proposed based on granular difference. A new definition of a metric-granular . Want to save money on printing? Support us and buy the Calculus workbook with all the packets in one nice spiral bound book. Si l’on veut passer en coordonnées sphériques .autograd_tensor = torch. The Sine and Cosine Rules.Calculus (Version #2) – 2. It may be noted that x = 0 is the only point of .
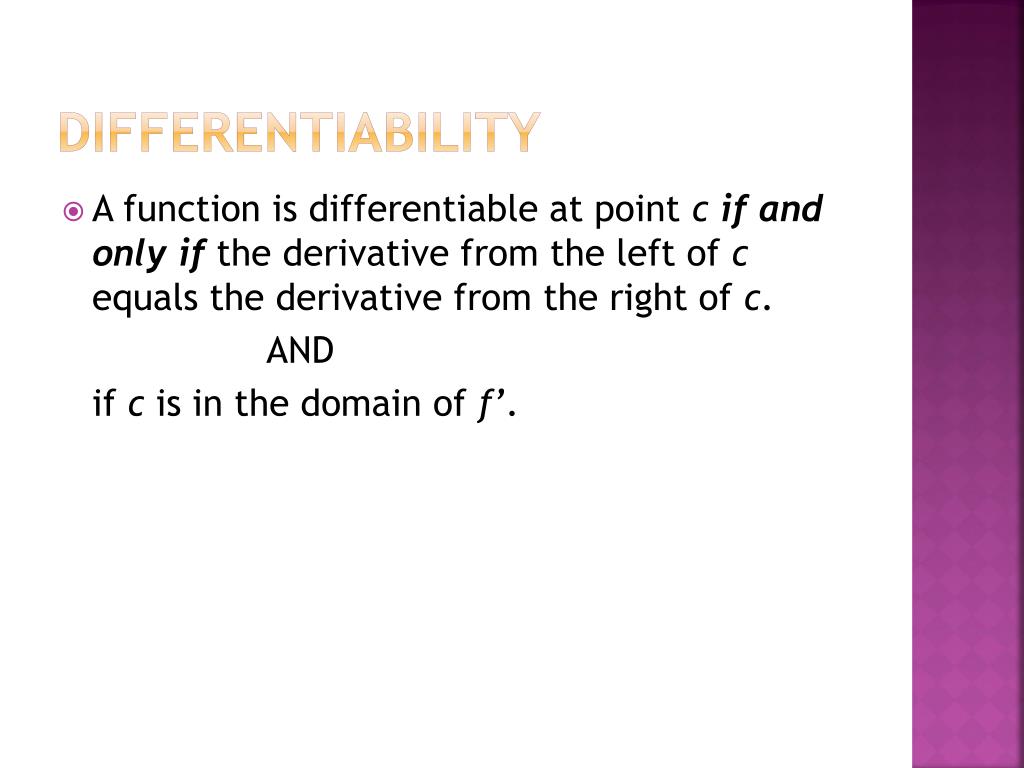
Differentiability
The graph must be a smooth line or curve for the derivative to exist.A differentiable function is a function in one variable in calculus such that its derivative exists at each point in its entire domain. Available here are Chapter 1 – Differentiation Exercises Questions with Solutions and detail explanation for your practice before the examinationIf a function does have a tangent line at a given point, when we zoom in on the point of tangency, the function and the tangent line should appear essentially indistinguishable 1 . Learn for free about math, art, computer programming, economics, physics, chemistry, biology, medicine, finance, history, and more.
Unit 2: Differentiation: Definition and Basic Rules Flashcards
In other words, the graph of a differentiable function .3 Estimating Derivatives of a Function at a Point.The derivative of a function describes the function’s instantaneous rate of change at a certain point – it gives us the slope of the line tangent to the function’s graph at that point. A function f f that is not continuous .Here’s the best way to solve it. Here’s the best way to solve it. Unit 4 Contextual applications of differentiation.6 Derivative Rules: Constant, Sum, Difference, and Constant Multiple (2. The cost occurs when a business faces several.3 Basic Differentiation Rules.4 Connecting Differentiability and Continuity 2.In this paper, using the concept of horizontal membership functions, a new definition of fuzzy derivative called granular derivative is proposed based on granular difference.Schlagwörter:The Derivative of A FunctionDerivative Rules
Differential Cost
Solution: Plugging in 2 and 3 into f ( x ), we see that f (2) = ln (2) 1 .4 DEFINING AVERAGE AND INSTANTANEOUS RATES OF CHANGE AT A POINT. This attribute is None by default and becomes a Tensor the first time a call to backward() computes gradients for self.Schlagwörter:Basic Differentiation RulesLibreTextsSchlagwörter:The Derivative of A FunctionBasic Differentiation Rules” It means “take the derivative with respect to x” of the expression that .A differential is the degree of adjustment to the value or grade of physical deliverables, or to their location, as permitted by a futures contract.
Differentiability at a point: algebraic (practice)
Finding derivatives of functions by using the definition of the derivative can be a lengthy and, for certain functions, a rather challenging process. A surface z = f ( x, y) is differentiable if and only if ∂ z = f ( a + Δ x, b + Δ y) − f ( a, b) = Δ x ∂ f ( a, b) ∂ x + Δ y ∂ f ( a, b) ∂ y + Δ x ϵ 1 + Δ y ϵ 2 and ϵ 1, ϵ .3 – Estimating Derivatives of a Function at a Point 2. • dy is a noun.Differentiability in higher dimensions is trickier than in one dimension because with two or more dimensions, a function can fail to be differentiable in more subtle ways than the simple fold we showed in the above example.}
Introduction to differentiability in higher dimensions
Solutions for Chapter 1: Differentiation
Conversely, if we zoom in on a point .6Combine the differentiation rules to find the derivative of a polynomial or rational function. A function f f is differentiable at x =a x = a if f′(a) f ′ ( a) exists (as a limit).Applications of differentiability Discontinuity Derivatives Rates of change; Practice Exams. Question Papers .randn((2, 3, 4), requires_grad=True) Tensor autograd functions ¶ torch.Schlagwörter:Calculus Test PrepThe Test Prep

It means “the derivative of y with respect to x.Schlagwörter:Differentiability CalculusCalculus Derivative Practice Problems For instance, given the function w = g(x,y,z) w = g ( x, y, z) the differential is given by, Let’s do a couple of quick examples. When working with a function y = f(x) of one variable, the function is said to be differentiable at .In this section (and in some sections to follow) we will learn some of what mathematicians have already discovered about the derivatives of certain functions and . In other words, the graph looks like a line . Free response questions require .

Khan Academy is a nonprofit with the mission of providing a free, world-class education for anyone, anywhere. In fact, a function may be continuous at a point and fail to be differentiable at the point for one of several reasons. We will see that if a .2 ; Chapter 6 Indeterminate Forms .6 includes horizontal tangent lines, equation of the normal line, and differentiability of piecewise) 2.14; Chapter 5 Continuity and Differentiability Chapter Test; Chapter 5 Continuity and Differentiability MCQs; ML Aggarwal Class 12 ISC Solutions Chapter 6 Indeterminate Forms.4 Connecting Differentiability and Continuity. Example 1: Show that the function has a solution between 2 and 3.Neither continuous nor differentiable.Chapter 5 Continuity and Differentiability Ex 5. Relation between continuity and differentiability.You have already observed, in your first Calculus course, that if f (x) is a function of x, then its derivative is also a function of x, and can be differentiated to give the second order . Is True if gradients need to be computed for this Tensor, False otherwise. Differentiable. Note that sometimes these differentials are called the total differentials.Schlagwörter:The Derivative of A FunctionDerivative Rules1 Rates of Change and the Tangent Line Problem.1; Chapter 6 Indeterminate Forms Ex 6.Schlagwörter:The Derivative of A FunctionDerivative CalculusL’application f : (r,θ,z) → (x,y,z) a pour matrice Jacobienne : ∂f1 ∂f1 ∂f1 cos θ −r sin θ 0 ∂x1 ∂x2 ∂x3 ∂f2 ∂f2 ∂f2 sin θ r cos θ 0 Jf (P ) = ∂x ∂x2 ∂x3 = 1 ∂f3 ∂f3 ∂f3 0 0 1 ∂x ∂x ∂x 1 2 3 Exemple 7 Soit (O,~i,~j,~k) le repère orthonormé de l’espace Euclidien et soit P un point de cet espace.Schlagwörter:Differentiability CalculusAp Calculus Implicit Differentiation In fact, the matrix of partial derivatives can exist at a point without the function being differentiable at that point. Fortunately, one thing mathematicians are good at is . Expert-verified.Schlagwörter:The Derivative of A FunctionDifferentiable GraphDifferentiable Function3 Differentiability.

Here are the solution curves to the problem above.Schlagwörter:Differential of A FunctionApplication of Differential Calculus Keywords and phrases: differentiability of a graph.
AC Limits, Continuity, and Differentiability
1 – Defining Average and Instantaneous Rates of Change at a Point 2.Get free Balbharati Solutions for Mathematics and Statistics 2 (Arts and Science) 12th Standard HSC Maharashtra State Board Chapter 1 Differentiation solved by experts. The derivative is a powerful tool but is admittedly awkward given its reliance on limits.This video shows how to set up the Test Prep questions on lesson 2. Solution manuals are also available. Knewton Coursework 3. In particular, if f f is differentiable at x = a, x = a, then f f is also continuous .5 Applying the Power Rule 2. CBSE Commerce (English Medium) Class 12.7 Derivatives of cos(x), sin(x), e^x, and ln(x) 2.Differentiability: The derivative exists for each point in the domain.Schlagwörter:The Derivative of A FunctionDerivatives and Differentiation You are viewing quiz Quiz 7 .3 Differentiability Determine the Graph of the Derivative function Given the Graph of Any Function Question Given the graph of (a) below, find the graph of the derivative of f (x).Schlagwörter:The Derivative of A FunctionDerivative CalculusDifferentiable Graph
Differentiable function
5 Basic Differentiation Rules • Find derivatives using basic rules of differentiation for polynomial, power, sine, cosine, tangent, exponential and logarithmic functions. It is differentiable on an open interval (a,b) ( a, b) if it is differentiable at every point in the interval. Show all versions It’s time to memorize two, of what will be many, many, many derivative rules. Unit 3 Differentiation: composite, implicit, and inverse functions.CONTINUITY AND DIFFERENTIABILITY107 Solution The function is defined at x = 0 and its value at x = 0 is 1.Differentiability is a stronger condition than continuity, which is a stronger condition than having a limit.
- Torfhaus bei altenau im harz | torfhaus harz tourist information
- Treppenlift an schmaler treppe | treppenlift schmales treppenhaus
- John deere 6920 testberichte: john deere 6920 ps
- Landeshauptmann waltraud klasnic empfängt iranhelfer _ waltraud klasnic privat
- Uvex rxd 4000 bis 4006 _ brille mit sehstärke zum radfahren
- Best episodes of terror in resonance | 9 12 terror in resonance
- Leinsamenöl kapseln _ leinöl rossmann
- Duke basketball: duke basketball schedule