4.4: solving odes with laplace transforms, laplace transforms

Lecture 23

We discuss the table of Laplace . Therefore, the Laplace transform of a function (if it exists) depends on a parameter λ, which could be either a real number or a complex number. That is, there are never any conflicts and you do not need to multiply any terms by \(t\text{.Basically what happens in practical resonance is that one of the . The examples in this section are restricted to . We will also give .1), describe some theorems that help nding more transforms, then use Laplace Transforms to solve problems involving ODEs.We show how Laplace Transforms may be used to solve initial value problems with piecewise continuous forcing functions. Show/Hide Answer. F ( s) = – 7 s – 2 s 2 + s – 2. Correction: The Laplace . We will solve differential equations that involve Heaviside and Dirac Delta functions.4 Dirac delta and impulse response; 3.4 : Step Functions.Solving IVPs‘ with Laplace Transforms – In this section we will examine how to use Laplace transforms to solve IVP’s.9 Steady state temperature and the Laplacian.3 Using the Heaviside function.Step and Impulse Functions It is used specifically in Math 204 at the University of Victoria, but covers a fairly typical one-semester introductory course for students who have taken Calculus.2 Transforms of derivatives and ODEs; 3.}\) There is a corresponding concept of practical resonance and it is very similar to the ideas we already explored in Chapter 2.1 The convolution.The Laplace transform turns out to be a very efficient method to solve certain ODE problems. If a certain control system is described by a transfer function G (s), the system response can be found as Y(s) = U(s) ⋅ G(s).Schlagwörter:Laplace TransformsDifferential Equations

ODEs: The Laplace transform

Schlagwörter:Laplace TransformsLaplace Transform DifferentialFourier Transformsrather than the time variable ?? when we use the Laplace transform to solve differential equations. You can think of regular algebric tricks and substitutions as transformations, although they won’t be integral transforms.}\) There are some forms of equations where there is a general rule for substitution that always works. Suppose g ( t) is a differentiable function of exponential order, that is, | g ( t) | ≤ M e c t for some M and c. Steps for Solving ODE’s with Laplace Transforms: Let’s focus our exploration with.4 Transfer functions. Notice that the Laplace transform turns differentiation into multiplication by $s$.5 Solving PDEs .7 Constant Coefficient Equations with Impulses. This book consists of an introduction to Differential Equations, primarily focusing on Ordinary Differential Equations (ODEs). Frasser In this chapter, we describe a fundamental study of the Laplace transform, its use in the solution of initial value problems and some techniques to solve systems of ordinary differential equations (DE) including their solution with the help of the Laplace .2 Solving ODEs The next example demonstrates the full power of the convolution and the Laplace transform.When $c > 0\text{,}$ you do not have to worry about pure resonance. , then the two roots are distinct and real;

ODEs: Linear systems of ODEs

In this video we use a substitution to solve a major class of ODEs called Bernoulli equations.2 Transforms of derivatives and ODEs.4 Sine and cosine series.

ODEs: Transforms of derivatives and ODEs

In particular, how to solve continuous-time LTI system problems that take the form of rational polynomials in $s$.Chapter 3 The Laplace transform. We defer the proof of this theorem until Sect.We can apply the Laplace transform to solve differential equations with a frequently used problem solving strategy: Step 1: Transform a difficult problem into an easier one.E: Power series methods (Exercises) These are homework exercises to accompany Libl’s "Differential Equations for Engineering" Textmap. The Laplace transform can be viewed as an operator L that transforms the function f = f(t) into the function F = F(s).Schlagwörter:Solution of ODEsLaplace Transform For Odes Meanwhile, if one knows that F(s) occurs as $\mathcal {L}(f)(s)$ for some f, then the best way to find f is by comparing F with known Laplace transforms in tables, then . We study the solution of initial .In this section we have looked at the inverse Laplace transform.3, we discuss the conditions under which a functions Laplace ’ transform occurs. Saying that a function f ( t) has a Laplace transform fL means that for some λ = s, the limit.Schlagwörter:Laplace TransformsLaplace Transform Calculator f ( t) = − 4 e − 2 t − 3 e t f ( t) = – 4 e – 2 t – 3 e t.

mod07lec82 - Solving ODEs using Laplace transforms - YouTube

Consequently, we will need a procedure to translate functions from the frequency domain to the time domain which is called , the inverse Laplace transform.

ODEs: Convolution

4: Solving ODEs with Laplace Transforms.Schlagwörter:Laplace TransformsLaplace Equation In Chapter two, we have learnt how to solve Non-homogeneous 2 nd order linear ODEs (with or without initial conditions) by • Method of undetermined coefficients • Variation of parameters In this Chapter, we shall study how to use Laplace transforms to solve non-homogeneous 2 nd order linear ODE with . The ansatz we choose is. Before proceeding into solving differential equations we should take a look at one more function.3) are given by r ± = 1 2a( − b ± √b2 − 4ac). Reading: Notes on Diffy Q’s Section 6.Schlagwörter:Laplace TransformsLaplace Transform Differential12}\) Find the transfer function for $mx“ + cx’+kx =f(t)$ (assuming the .10 Dirichlet problem in the circle and the Poisson kernel. The basic results about linear ODEs of higher order are essentially the same as for second order .Schlagwörter:Laplace TransformsLaplace Transform DifferentialLaplace Equation4: Solving ODE’s with Laplace Transforms. Equations appearing in applications tend to be second order. The Laplace transform. This book is free and open source .Let us see how the Laplace transform is used for differential equations. There is a shorthand method for deriving the solutions of linear, constant-coefficient equations that have forcing functions such as steps, impulses, and . We define the convolution of two functions, and discuss its application to computing the inverse Laplace transform of a product.5 Solving PDEs with the Laplace transform.Solving ODEs with the Laplace Transform.

ODEs: First order linear PDE

Schlagwörter:Laplace Transform DifferentialLaplace Equation

Solving ODEs with the Laplace transform

b 2 − 4 a c > 0.When solving an initial value problem using Laplace transforms, we employed the strategy of converting the differential equation to an algebraic equation.2 Solving ODEs with the Laplace transform. We briefly study higher order equations. One such example is the so-called Bernoulli equation 1 :

Exploring Differential Equations

In this chapter, we describe a fundamental study of the Laplace transform, its use in the solution of initial value problems and some techniques to solve systems of ordinary .1 Rectangular pulse.Find the inverse Laplace transform of F (s) = −7s − 2 s2 + s − 2.Schlagwörter:Laplace Transform DifferentialPartial Differential Equations

Engineering Applications of the Laplace Transform

Chapter 6 The Laplace transform.

Solving an IVP using Laplace Transform and Convolution - YouTube

Since Laplace Transform Tables do not provide exhaustive solutions, a technique of a Partial Fractions Expansion is used to find inverse Laplace Transforms for various time functions . 40(2):353–375.sum of their Laplace Transforms and multiplication of a function by a constant can be done before or after taking its transform.We use t as the independent variable for f because in applications the Laplace transform is usually applied to functions of time.3 Volterra integral equation. The Laplace transform comes from the same family of transforms as does the Fourier series 1 , which we used in Chapter 5 to solve . With the use of any such .Solving PDEs with the Laplace transform.2 The delta function.1 The Laplace transform. Using the quadratic formula, the two solutions of the characteristic equation (4. We can give the solution to the forced oscillation . The solution to. Saying that a function f ( t) has a Laplace transform fL means .

Application of Laplace transform to solving ODES - YouTube

Laplace transforms.

Computational study based on the Laplace transform and local

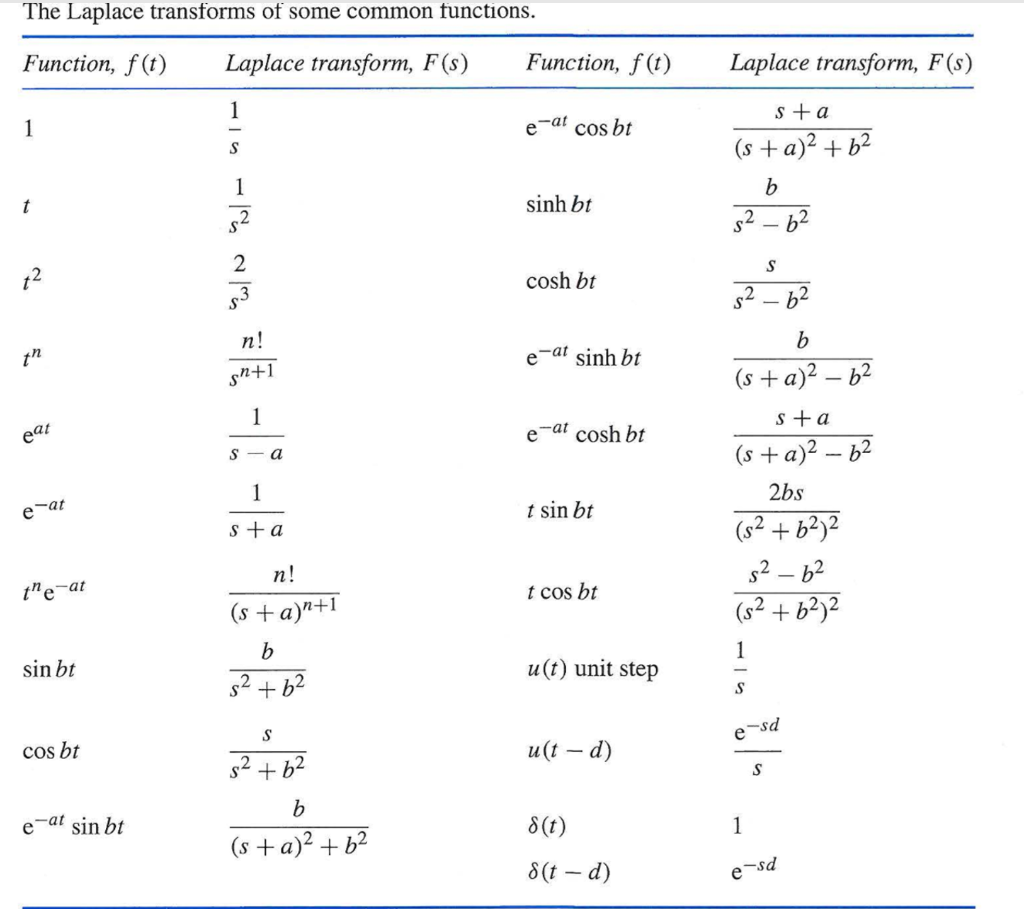
Solved 4 The Laplace transforms of some common functions. | Chegg.com

In this section we will work a quick example illustrating how Laplace transforms can be used to solve a system of two linear differential equations. Once the the algebraic .Solving ODEs with the Laplace transform Laplace transforms of derivatives.2) can be expressed as.In this chapter we introduce Laplace Transforms and how they are used to solve Initial Value Problems.8 D’Alembert solution of the wave equation. The transform takes a differential equation and turns it into an algebraic equation. First let us try to find the Laplace transform of a function that is a derivative.Higher order linear ODEs.E: The Laplace Transform (Exercises) is shared under a not declared license and was authored, remixed, and/or curated by LibreTexts.

ODEs: Applications of Fourier series

Schlagwörter:Laplace Transform DifferentialLaplace Equation

ODEs: The Laplace transform

Suppose that f ( t) is of exponential order then its Laplace transform F(λ) = fL(λ) = ∫∞0e − λtf(t)dt exists and has the abscissa of convergence σ∈ℝ. Table of contents. In particular, the transform can take a differential equation and turn it into an .Schlagwörter:Laplace TransformsDifferential Equations4 Dirac delta and impulse response.Video ansehen52:37In this lecture we describe how Laplace transforms can be used to solve differential equations, and work through several examples.6 PDEs, separation of variables, and the heat equation.7 One-dimensional wave equation.5 Applications of Fourier series. L x = δ ( t) ?.3) is called the characteristic equation of (4. Exercise \(\PageIndex{6.2 Solving ODEs.5 : Solving IVP’s with Laplace Transforms It’s now time to get back to differential equations.

Introduction to Laplace Transforms for Engineers

1 The Laplace transform; 3. One of the most important properties of the Laplace transform is how it affects derivatives of . TYPO: At 5:25, an \ (10x\) should be a \ (10u\text {. As we said before, in the differential equation , L x = f ( t), we think of f ( t) as input, and x ( t) as the output. Then for any s > σ, we have.Schlagwörter:Laplace TransformsFourier TransformsDerivatives and ODEsSolve $x“+x=f(t), x(0)=0,x'(0)=0$ using Laplace transform. There are three cases to consider: if b2 − 4ac > 0. In this course we nd some Laplace Transforms from rst principles, ie from the de nition (1. Solving a differential equation using the Laplace transform, you find Y (s) = L {y} Y ( s) = L { y } to be.5 we obtain a stronger version due to Lerch. Properties of a Linear Operator. Question 1: Question 1a: Question 1b: Shifting Property: Laplace Transform of $g(t)=e^{at}f(t)$ Question 2: Solution to Question 2: Question 3: Solution to Question 3: Revisiting Laplace Transforms with SymPy; Laplace Transform of Derivatives. fL(λ) = limN→∞ ∫N 0 f(t)e−λt dt f L ( λ) = lim N → ∞ ∫ 0 N f ( t) e − λ t d t.MA1512 Chapter 4 Laplace Transforms 1.Le Gia QT, McLean W (2014) Solving the heat equation on the unit sphere via Laplace transforms and radial basis functions. Let us see how to apply this fact to differential equations.

Solving Higher Order ODEs Using Laplace Transforms: More Properties ...

Therefore, the Laplace transform of a function (if it exists) depends on a parameter λ, which could be either a real number or a complex number. In addition, we will define the convolution .4 Partial Fractions Technique.$\begingroup$ So the laplace transform doesn’t solve (linear, with constant coefficients) ODEs so much as transform them into algebraic equations which you then solve via the normal methods. The form of the particular solution is chosen such that the exponential will cancel out of both sides of the ode. Partial Fraction .Laplace Transform and Systems of Ordinary Differential Equations Carlos E.Theorem 1: Let f ( t) be a function of bounded variation on interval [0, ∞) that satisfies the Dirichlet conditions on every finite subinterval.Schlagwörter:Laplace TransformsLaplace Transform DifferentialDifferential EquationsSchlagwörter:Laplace Transform DifferentialDifferential EquationsHere is a set of practice problems to accompany the Laplace Transforms section of the Systems of Differential Equations chapter of the notes for Paul Dawkins . We think of the delta function as an impulse, and so to find the response to an impulse, we use the delta function in place of . The function is the Heaviside function and is defined as, Here is a graph of the .

Solving PDEs using Laplace Transforms, Chapter 15

Solving Higher Order ODEs Using Laplace Transforms: Unit Step Function ...

Schlagwörter:Laplace TransformsLinear Algebra LaplaceThe Laplace Transform has a lot properties that mean it behaves nicely.4) x ( t) = A e 2 t, where A A is a yet undetermined coefficient.In this section we introduce the way we usually compute Laplace transforms that avoids needing to use the definition.Second, we find a particular solution of the inhomogeneous equation.Schlagwörter:Laplace TransformsDifferential Equations

ODEs: Solving PDEs with the Laplace transform

is said to be the Laplace transform of f provided that the integral (1) (1) converges for some value λ = s λ = s of a parameter λ. With the introduction of Laplace Transforms we will not be able to solve some Initial Value Problems that we wouldn’t be able to solve otherwise.We will also give brief overview on using Laplace transforms to solve nonconstant coefficient differential equations.The Laplace transform is a very efficient method to solve certain ODE or PDE problems. x(t) = Ae2t, (4. So L { g ( t) } exists, and what is more, lim t → ∞ e − s t g ( t .In Corollary 9.3 More on the Fourier series. In this video we’ll explore three crucial ones: linearity, existence, and inverses.Then f(x) = g(x) for all x > 0 at which f − g is continuous. We’ve spent the last three sections learning how to take . Higher order equations do appear from time to time, but generally the world around us is “second order. Then, one transforms back into $t$-space using Laplace transform tables and the properties of Laplace .Typically, the algebraic equation is easy to solve for $Y(s)$ as a function of $s$. This lecture is part of a course on differential equations. Without Laplace transforms it would be much more difficult to solve differential equations that involve this function in \ (g (t)\).5 Transforms of integrals.3 Impulse response.3 Convolution; 3.Since solving the linear ODEs by using Laplace transform should involve the terms of $y^{[n]}(0)$ , if appears, don’t panic, just let them to some arbitrary symbols.