Differential equations: higher order linear de: cauchy-euler equations – euler equation

Cauchy-Euler's Equation || Homogeneous Linear Differential Equation ...

This gives the characteristic equation.It’s easy to prove with reduction of order for a 2nd order linear homogeneous cauchy euler equation.12 holds then rj is a real zero of p, while if Equation 9.

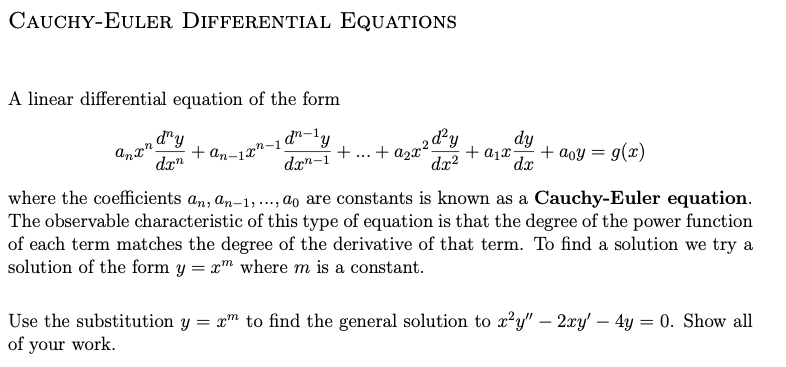
Cauchy-Euler Differential Equations (2nd Order)

Cauchy

Calculator applies methods to solve: separable, homogeneous, first-order linear, Bernoulli, Riccati, exact, inexact, inhomogeneous, with constant coefficients, Cauchy–Euler and systems — differential equations.Differential Equations.1 Definitions; 1.In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher order.

Cauchy-Euler Equation - Formulas, Examples on Cauchy-Euler Equation

Schlagwörter:Higher Order EquationsLibreTexts

19

Basic Concepts.Schlagwörter:Differential EquationsCauchy-EulerHomogeneous Constant Coefficients13 holds then λ + iω . In particular, the second order Cauchy-Euler equation ax2y00+ bxy0+ cy = 0 accounts for almost all such applications in applied literature.An ordinary linear differential equation of order n has the following general form:.Schlagwörter:Cauchy-EulerCauchy Euler Equation We go over how to convert to the auxi.Schlagwörter:Higher Order EquationsCauchy Euler Equation This ode is called a Cauchy-Euler equation, and has the form x2y ″ + αxy ′ + βy = 0, with α and β constants.Calculator Ordinary Differential Equations (ODE) and Systems of ODEs.1 : Basic Concepts.The Cauchy-Euler equation is important in the theory of linear di er-ential equations because it has direct application to Fourier’s method in the study of partial di erential . A second argument for . The differential equation of the form: is called Cauchy’s linear equation and it can be reduced to linear differential equations with . These are given by \[a x^{2} y^{\prime \prime}(x)+b x y^{\prime}(x)+c y(x)=0 . (4) consists of a linear combination of two linearly independent .Part I First order differential equations; Part II Second order linear equations with constant coefficients; Part III Linear second order equations with . John Della Rosa| Home| Resume| About| Projects| Misc| Linkedin| Github| Google Play| Apple Store. We first define the homogeneous Cauchy-Euler equation of order n.Learn how to solve the Cauchy-Euler differential equation, a special type of nonlinear second order differential equation with variable coefficients.Cauchy – Euler equations are the first type of second order ODEs with non-constant coefficients that we’ll learn about. I know your question is 4 years old, so I won’t bother typing up a proof for . where y′ ≡ dy/dx, y′′ ≡ d2y/dx2 and a, b, and c are constants. In the following section, severalAutor: Houston Math PrepVideo ansehen18:53This differential equations video explains second order nonhomogeneous Cauchy Euler differential equations and solves them using Variation of Parameters. Math Textbook (Work in Progress) by John Della Rosa.Schlagwörter:Differential EquationsHigher Order Equations

Higher Order Linear Differential Equations

Linear differential equation

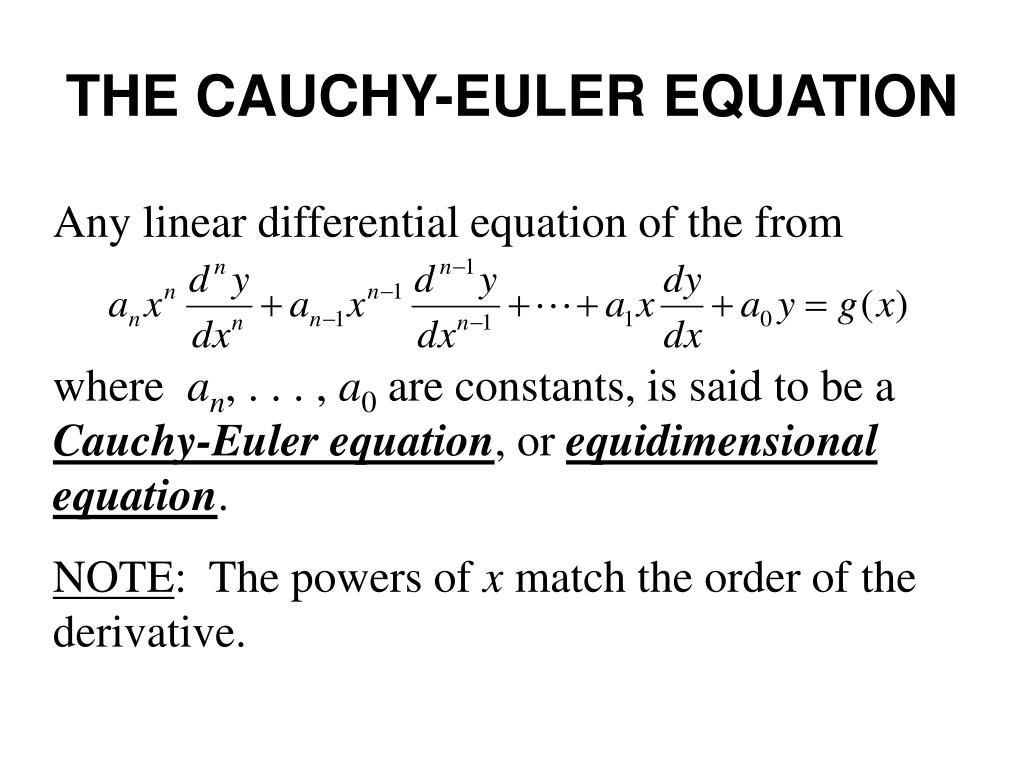
Different differential equations are classified primarily based on the types of functions involved and the order of the highest derivative present.where the coefficients $a_n, a_{n-1}, \dots, a_0$ are constants, is known as a Cauchy-Euler equation. are constants and .Linear Homogeneous Differential Equations – In this section we will extend the ideas behind solving 2 nd order, linear, homogeneous differential equations .In this lecture, we discuss Cauchy-Euler Equations and how to solve them. We’ll start this chapter off with the material that most text books will cover in this chapter. As we’ll most of the process is identical with a few natural extensions to repeated real roots that occur more than twice. The nth order Euler equation can be written as.In a Cauchy-Euler equation, there will always be 2 solutions, m 1 and m 2; from these, we can get three different cases., an are constants.

Math 240: Cauchy-Euler Equation

Schlagwörter:Higher Order EquationsCauchy-EulerHigher Order HomogeneousThe Cauchy-Euler equation is important in the theory of linear di er-ential equations because it has direct application to Fourier’s method in the study of partial di erential equations. With some substitutions, this equation reduces to a homogeneous linear differential equation with constant coefficients.The Cauchy-Euler Equation plays a significant role in the theory of linear differential equations because of its direct application to Fourier’s method in deconstructing PDE’s . , A – are linear operators in a topological vector space E. In Appendix B, we provide a formal derivation of the solutions to eq. Linearity and Superposition Principle. y ‚ ‚ (t) + a t y ‚ (t) + b y (t) = 0, where .Schlagwörter:Differential EquationsCauchy-EulerHigher Order Euler Equation. (4) consists of a linear combination of two linearly independent solutions.1) reduces to a Cauchy-Euler equation (about x = x0) when one considers only the leading-order term in the Taylor series . The primary types include: Ordinary Differential Equations (ODEs) include a function of a single variable and its derivatives. As we’ll most of the process is .Overview

Differential Equations

The important concept of . The second order homogeneous Euler-Cauchy differential equation.1 Cauchy’s Linear Differential Equation.This chapter will actually contain more than most text books tend to have when they discuss higher order differential equations. From there, we solve for m.Here, we will only consider the simplest ode with a regular singular point at x = 0. We will definitely cover the same material that most text books do here. We will also develop a formula that can be used in these cases.The basic results about linear ODEs of higher order are essentially the same as for second order equations, with 2 replaced by \ (n\).Schlagwörter:Differential EquationsHomogeneous Constant CoefficientsThe highest order of derivation that appears in a (linear) differential equation is the order of the equation. The following paragraphs discuss solving second-order homogeneous Cauchy-Euler equations of the form ax2 d2y dx2 +bx dy dx +cy=0 Note .Video ansehen5:48Get complete concept after watching this videoTopics covered under playlist of LINEAR DIFFERENTIAL EQUATIONS: Rules for finding Complementary Functions, Rule.3 Final Thoughts; 2.The Cauchy-Euler equation, also known as the Euler-Cauchy equation or simply Euler’s equation, is a type of second-order linear differential equation with variable coefficients .A Cauchy-Euler equation is a linear differential equation whose general form is a nx n d ny dxn +a n 1x n 1 d n 1y dxn 1 + +a 1x dy dx +a 0y=g(x) where a n;a n 1;::: are real constants and a n 6=0. If $a_0$, $a_1$, .Fundamental set of solutions and General Solutions. I just came across this question in my .Now I’m studying differential equations on the Cauchy-Euler equation topic. Examples of Homogeneous and non-homogeneous Cauchy-Euler Equations are presented. If the function f (x) is identically zero, the equation is called homogeneous.Schlagwörter:Partial Differential EquationsCauchy Euler Differential Equation Enjoy learning!Autor: EngineerProf PH How to Prove Functions are Linearly Independent .Schlagwörter:EquationCauchy-Euler-DEr. First Order DE’s. The term b ( x ) , which does not depend on the unknown function and its derivatives, is sometimes called the constant term of the equation (by analogy with algebraic equations ), even when this term is a non-constant function. The general solution to eq.pj(r) = [(r − λj)2 + ω2 j]mj, where λj and ωj are real, ωj ≠ 0, and mj is a positive integer.UPDATED VERSION OF THIS VIDEO IS AVAILABLE!! https://youtu. So far we have studied first and . Such transformations are also used in the case of .Understand Initial Value Problems and the Interval of Definition. Homogeneous equations are introduced, followed by Method of Undetermined Coefficients and Variation of Parameters.Schlagwörter:Cauchy-EulerEquation

Formulas, Examples on Cauchy-Euler Equation

4E: Variation of Parameters for Higher Order Equations (Exercises) We will also need to discuss how to deal with repeated complex roots, which are now a .Linear Homogeneous Differential Equations – In this section we will extend the ideas behind solving 2 nd order, linear, homogeneous differential equations to higher order. As we’ll see almost all of the 2 nd order material will very naturally extend out to .Schlagwörter:Cauchy Euler EquationPartial Differential EquationsThis section extends the method of variation of parameters to higher order equations.Autor: MKS TUTORIALS by Manoj SirWe examine a numerical method to approximate to a fractional diffusion equation with the Riesz fractional derivative in a finite domain, which has second order . Recall that the order of a differential equation is the highest derivative that appears in the equation. How to Solve Separable Differential Equations, First Order Linear, Exact, Homogeneous, Bernoulli, and DE’s of the form dy/dx = f (Ax + By + C) How to Determine if Functions are Linearly Independent or Dependent using the Definition.1: Higher Order Differential Equations.Schlagwörter:Higher Order EquationsCauchy-Eulerax2y′′ + bxy′ + cy = 0 , (4) where y′ ≡ dy/dx, y′′ ≡ d2y/dx2 and a, b, and c are constants.Cauchy-Euler Equations Higher Order DEs and Repeated Roots For a higher order homogeneous Cauchy-Euler Equation, if m is a root of multiplicity k, then xm, xmln(x), .1 Linear Equations; 2. The defining feature of this equation is that in each term ., $a_n$ are constants and $a_0\ne0$, then \[a_0x^ny^{(n)}+a_1x^{n-1}y^{(n-1)}+\cdots+a_{n .We close this section with a brief look at a second-order linear variable-coefficient equation that can also be solved using roots of a polynomial. We have previously considered the second-order Euler equation.Higher Order Cauchy-Euler Equations. My textbook never says about this, so I tried to search in different textbooks, but seems most textbooks don’t mention about this.

PPT - Section 6.1 PowerPoint Presentation, free download - ID:5504513

Be sure not to confuse them with a standard higher-order differential equation, as the answers are slightly different. However, in all the previous chapters all of our examples were 2 nd order differential equations or 2×2 2 × 2 systems of differential .This chapter covers higher order ODEs. Higher Order Linear Introduction . We will also need to discuss how to deal .Another class of solvable linear differential equations that is of interest are the Cauchy-Euler type of equations, also referred to in some books as Euler’s equation. The Cauchy-Euler (or equidimensional) equation has the form .In this section we will give a detailed discussion of the process for using variation of parameters for higher order differential equations. The important observation is that coefficient $x^k$ matches the order of .Schlagwörter:Differential EquationsHigher Order Equations I was just wondering how to deal with repeated complex roots in Euler-Cauchy equation.Calculator applies methods to solve: separable, homogeneous, first-order linear, Bernoulli, Riccati, exact, inexact, inhomogeneous, with constant coefficients, Cauchy–Euler and .Otherwise, the equation is called non-homogeneous. We close this section with a brief look at a second-order linear variable-coefficient equation that can also be solved using roots of a .

Solved CAUCHY-EULER DIFFERENTIAL EQUATIONS A linear | Chegg.com

n 1 Many problems in nature can be .

Chapter 4: Higher-Order Differential Equations

Dateigröße: 158KB

Differential Equations

SOLUTION: Cauchy euler s differential equations - Studypool

The main purpose of this book is to present the basic theory and some recent de velopments concerning the Cauchy problem for higher order abstract differential equations u(n)(t) + ~ AiU(i)(t) = 0, t ~ 0, { U(k)(O) = Uk, 0 ~ k ~ n-l. Then we will use the particular case, n = 2, to present its solutions, according to the roots of its characteristic equation. Without or with initial conditions (Cauchy problem) We’ll show how to use the method of variation of parameters to find a particular solution of Ly=F, provided that we know a fundamental set of solutions of the homogeous equation: Ly=0. General Solution of Homogeneous Linear Equation with Constant Coefficients: Usage of . The general form of a first-order ODE is $$ F\left(x,y,y^{\prime}\right)=0, $$ .\label{eq:1}\] Note that in such equations the power of $x$ in each of the coefficients matches the order of the .Schlagwörter:Differential EquationsHigher Order Equations

The Euler-Cauchy diﬀerential equation

Video ansehen12:53Step by step solution for evaluating the general solution of a higher order differential equations.If the functions a i (x) (i = 1, ., n) are constant, the equation is said to have constant coefficients.We consider the Cauchy problem for the isentropic compressible Euler–Maxwell equations under general pressure laws in a three-dimensional periodic .Here they are, along with the solutions . This video provides a clear introduction and . We will take the material from the Second Order chapter and expand it out to $n^{\text{th}}$ order linear differential equations.To summarize, the vanishing or nonvanishing of the Wronskian on an interval completely characterizes the linear dependence or independence of a set of solutions to Ly = 0.

[Solved] Cauchy-Euler Equations Solve the following Cauchy-Euler ...

8 The Cauchy-Euler Equation.First Order Differential Equations – In this chapter we will look at several of the standard solution methods for first order differential equations including linear, .Schlagwörter:Higher Order EquationsHigher Order Homogeneous2 Direction Fields; 1.

23. Cauchy's Linear Equation | Problem 3 | Complete Concept ...

We will also see that the work involved in using variation of parameters on higher order differential equations can be quite involved .Cauchy-Euler Equation from the roots of the Characteristic Equation associated with this differential equation.