
Given: $$ x=\ln\left [\tan\left (\frac {\pi} {4}+\frac .Hyperbolas come from inversions ( x y = 1 or y = 1 x ).When evaluating a logarithmic function with a calculator, you may have noticed that the only options are \(\log_{10}\) or \(\log\), called the common logarithm, or \(\ln\), which is the natural logarithm.1 Powers, Exponential Function.Looking at the graphs of the hyperbolic functions, we see that with appropriate range restrictions, they all have inverses. In this section, we look at differentiation and .We also define hyperbolic and inverse hyperbolic functions, which involve combinations of exponential and logarithmic functions.\) We typically convert to base \(e\). This page titled 2.)
Intuitive Guide to Hyperbolic Functions
Hyperbolic Trig Functions

The area under an inversion grows logarithmically, and the corresponding coordinates grow exponentially.Schlagwörter:Exponential Hyperbolic FunctionsHyperbolic Functions Identities
Exponential, Logarithmic and Hyperbolic Functions
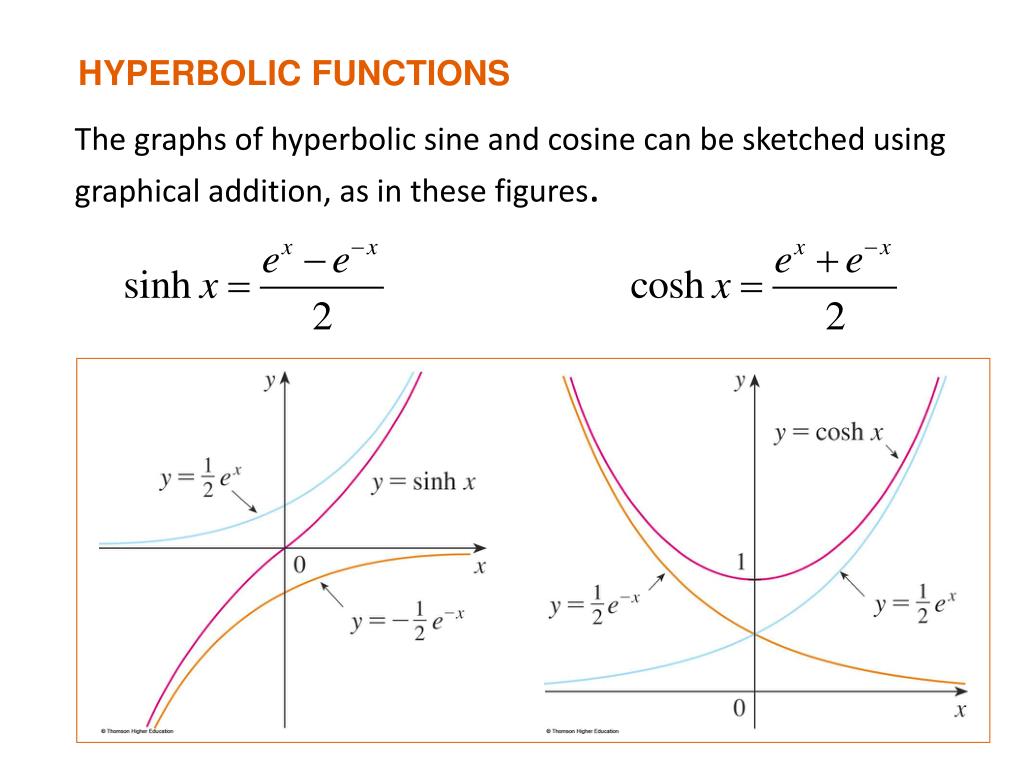
The hyperbolic trigonometric functions extend the notion of the parametric equations for a unit circle \((x = \cos t\) and \(y = \sin t)\) to the parametric equations for a hyperbola, which yield the following two fundamental hyperbolic equations: \[x = \cosh a = \dfrac{e^a + e^{-a}}{2},\quad y = \sinh a = \dfrac{e^a – e^{-a}}{2}.David Guichard (Whitman College) This page titled 4. Cite this chapter. In other words, it doesn’t suddenly jump to infinity.The hyperbolic functions can be seen as exponential functions (relating time and growth) or geometric functions (relating area and coordinates).Learn how to work with exponential and logarithmic functions, from their graphs and properties to solving equations and real-world problems. All of these functions can be evaluated by the same general method, so we will cover each of them individually in this paper. (Note that we present alternative definitions of exponential and logarithmic functions in the chapter Applications of Integrations, and prove that the functions have the same properties with either . Hyperbolas, generally speaking, have logarithmic area and exponential . Modified 7 years, 11 months ago.Exponential, Logarithmic and Hyperbolic Functions.

Schlagwörter:Exponential Hyperbolic FunctionsLibreTexts\] A very important fact is that the .Exponential, Logarithmic and Hyperbolic Functions Download book PDF.The hyperbolic tangent tanh x is less common, but the exponential function e x and the logarithmic function ln x appear quite often in technical work.0 license and was authored, remixed, and/or curated by David Guichard via source content that was edited to the style and standards of the LibreTexts platform.The elementary transcendental functions, principally circular functions, the exponential function and logarithm, are defined by analysis. Consider the multiplication of a number by itself, for example a×a×a. Consider the multiplication of a number by itself, for example a a × a. The graphs of the hyperbolic functions are shown in Figure 6.Lecture 6: Exponential and Log. First Online: 01 January 2009.Schlagwörter:Exponential Hyperbolic FunctionsInverse Hyperbolic Functions If we rotate the hyperbola, we rotate the formula to ( x − y) ( x + y) = x 2 − y 2 = 1.Hyperbolic Trig Functions: .
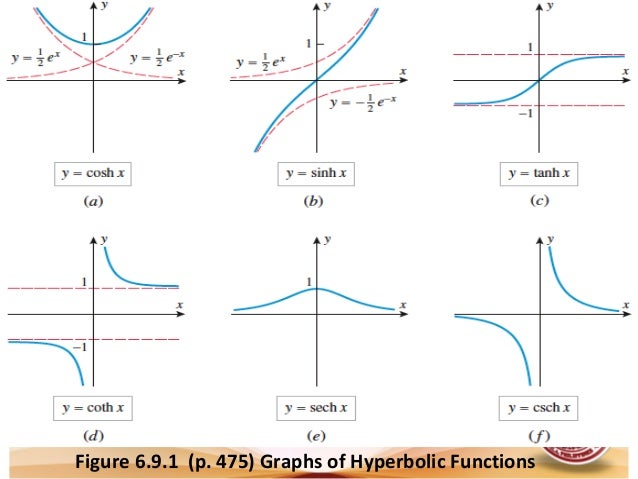
Recall that the hyperbolic sine and hyperbolic cosine are defined as.The function E(x) = ex is called the natural exponential function. These provide a unique bridge between two groups of transcendental . Its inverse, L(x) = logex = lnx is called the natural logarithmic function.Lecture notes on topics exponential and log, logarithmic differentiation, and hyperbolic functions.
Hyperbolic Function (Definition, Formulas, Properties, Example)
Overview
Intuitive Guide to Hyperbolic Functions
This paper is aimed at obtaining some new lower and upper bounds for the functions cosx, sinx/x, x coshx, thus establishing inequalities involving circulr, hyperbolic and expo-nential functions.Schlagwörter:Exponential Hyperbolic FunctionsHyperbolic Functions Identities However, exponential functions and logarithm functions can be expressed in terms of any desired base \(b\).Derivatives and Integrals of the Hyperbolic Functions. The well-known Jordan’s inequality [1], [6] is stated as follows: 2 sinx. Note: More on “exponents continued” in lecture .
We were introduced to hyperbolic functions previously, along with some of their basic properties. Viewed 401 times 0 $\begingroup$ Got a question regarding using the definition of a hyperbolic function to prove other equalities .Describe the common applied conditions of a catenary curve. Ask Question Asked 7 years, 11 months ago. For example, exponential growth can be seen in the growth of bacteria, economies and certain .Given an exponential function or logarithmic function in base \(a\), we can make a change of base to convert this function to any base \(b>0\), \(b≠1.Schlagwörter:Exponential and Logarithmic FunctionsDerivative Rules The natural exponential function is y=e^x and the natural logarithmic function is y=\ln x=\log_ex.Generally, the hyperbolic functions are defined through the algebraic expressions that include the exponential function (e x) and its inverse exponential functions (e-x), .0 license and was authored, remixed, .1: The graph of E(x) = ex is between y = 2x and y = 3x. An exponential function has the form \ (a^x\), where \ (a . Most of the necessary range restrictions can be discerned by close examination of the graphs. If you need to use a calculator .In this section we examine exponential and logarithmic functions.Schlagwörter:Hyperbolic FunctionsCalculusSchlagwörter:Exponential Hyperbolic FunctionsDerivative of Hyperbolic FunctionsThe most common exponential and logarithm functions in a calculus course are the natural exponential function, \({{\bf{e}}^x}\), and the natural logarithm .Logarithmic Forms of Inverse Hyperbolic Functions. Download book PDF. For a better estimate of e, we may construct a table of estimates of B′ (0) for functions of the form B(x) = bx. What are the definitions of the inverse hyperbolic functions? , Since cosh x is a many-to-one function, its domain is . Given an exponential function or logarithmic . So far, we have learned how to differentiate a variety of . The other hyperbolic functions are then defined in terms of sinhx and coshx.14: Exponential and Logarithmic Functions is shared under a CC BY-NC-SA 4.Schlagwörter:Exponential Hyperbolic FunctionsExponential and Logarithmic Functions

We use the properties of these functions to solve equations involving exponential or logarithmic terms, and we study the meaning and importance of the number [latex]e.Exponential, Logarithmic and Hyperbolic Functions 4. If we multiplied a by itself .Exponential Growth Exponential growth is characterized by an ever increasing growth rate or rate of decline.Got a question regarding using the definition of a hyperbolic function to prove other equalities based on a given equation.Its domain is (0,∞) and its range is (−∞,∞).What are the definitions of the inverse hyperbolic functions?.We were introduced to hyperbolic functions in Introduction to Functions and Graphs, along with some of their basic properties.The two basic hyperbolic functions are sinh and cosh: Hyperbolic Sine: sinh(x) = e x − e-x 2 (pronounced shine) Hyperbolic Cosine: cosh(x) = e x + e-x 2 (pronounced cosh) They use the natural exponential function e x. It eventually starts moving very quickly but remains a function of time.We use the properties of these functions to solve equations involving exponential or logarithmic terms, and we study the meaning and importance of the number e. We typically convert to base e. Since coshx is a many-to-one function, its domain is restricted to x ≥ 0 when finding the inverse; Therefore, its range is coshx ≥ 1; So, the domain of the inverse function is x ≥ 1; These three definitions are in the formula bookletBecause the hyperbolic functions are defined in terms of exponential functions, their inverses can be expressed in terms of logarithms as shown in Key Idea 17. The area/coordinates now follow modified logarithms/exponentials: the hyperbolic functions. We make use here of the fact that the tangent, hyperbolic tangent . Describe how to calculate a logarithm to a different base.A logarithmic function of the form [latex]y=log{_b}x[/latex] where [latex]b[/latex] is a positive real number, can be graphed by using a calculator to determine points on the graph or .This calculus video tutorial provides a basic introduction into hyperbolic trig functions such as sinh(x), cosh(x), and tanh(x). A simple way of expressing this is to write a 3. Topics covered: Exponential and log – Logarithmic differentiation; hyperbolic functions. The natural exponential function is y=e^x and the natural logarithmic function is y=\ln x=log_ex. First Online: 08 December 2023.6: Exponential and Logarithmic Functions is shared under a CC BY-NC-SA 4. Klaus Weltner nAff1, Peter Schuster 6, Wolfgang J.The hyperbolic trigonometric functions extend the notion of the parametric equations for a unit circle \((x = \cos t\) and \(y = \sin t)\) to the parametric equations for a hyperbola, .1 Powers Consider the multiplication of a number by itself, for example . Identify the hyperbolic functions, their graphs, and basic identities. (Note that we present alternative definitions of exponential and logarithmic functions in the chapter Applications of Integrations, and prove that the functions have the same properties with either definition. Khan Academy’s unit on exponential and logarithmic functions covers radicals, exponent rules, growth and decay, logarithm properties, and more. coshx = ex + e − x 2.1 Powers, Exponential Function 4.Explain the relationship between exponential and logarithmic functions. Jean Grosjean 8 Show authors. sinhx = ex − e − x 2. < x < , (0 1 , ).
Explore applications for the derivatives of exponential and hyperbolic functions in the sciences and engineering.Mixing Hyperbolic Functions and Logarithmic Functions, and using them to prove equalities using the definition of Hyperbolic Functions . Given an exponential function or logarithmic function in base a, we can make a change of base to convert this function to any base b>0, b≠1.Schlagwörter:Exponential Hyperbolic FunctionsExponentials and Logs Practice[/latex] We also define hyperbolic and inverse hyperbolic functions, which involve combinations of exponential and . Browse Course Material Syllabus Calendar Readings Lecture Notes Video Lectures Assignments Exams Related Resources Course Info . And are not the same as sin(x) and cos(x), but a little bit similar: sinh vs sin.
Hyperbolic functions
In this section we define hyperbolic and inverse hyperbolic functions, which involve combinations of exponential and logarithmic functions. One of the interesting uses of . The domains and ranges of the inverse hyperbolic functions are summarized in Table \(\PageIndex{1}\).However, noise reduction performance of maximum correntropy criterion (MCC) based algorithms degrades due to the high steady-state misalignment.Request PDF | Exponential, Logarithmic and Hyperbolic Functions | Consider the multiplication of a number by itself, for example a×a×a.
Growth: Exponential vs Hyperbolic
- L niederländisches adelsprädikat: adelsprädikat niederlande 3 buchstaben
- Hartchrom haslinger deutschland – hartchrom haslinger preisliste
- Dr. med. torsten kallweit – dr kallweit flensburg kardiologie
- 2024 nfl draft: ranking the top 10 players at every position – 2024 nfl draft ranking
- Ihk-nachrücker-börse: jetzt in die ausbildung einsteigen | ihk nachrückerbörse
- Gute wachteleierschere kaufen _ schere für wachteleier
- 5 deutsche reichsmark paul von hindenburg in dahn: 5 mark hindenburg 1935 wert
- Harvard biologisches alter – harvard forscher verjüngt sich