What is an example of a set of whole numbers?The set of whole numbers is the set of natural numbers with the addition of the number zero.The property that sets the real numbers apart from other sets of numbers, like the rationals, is a property known as completeness.
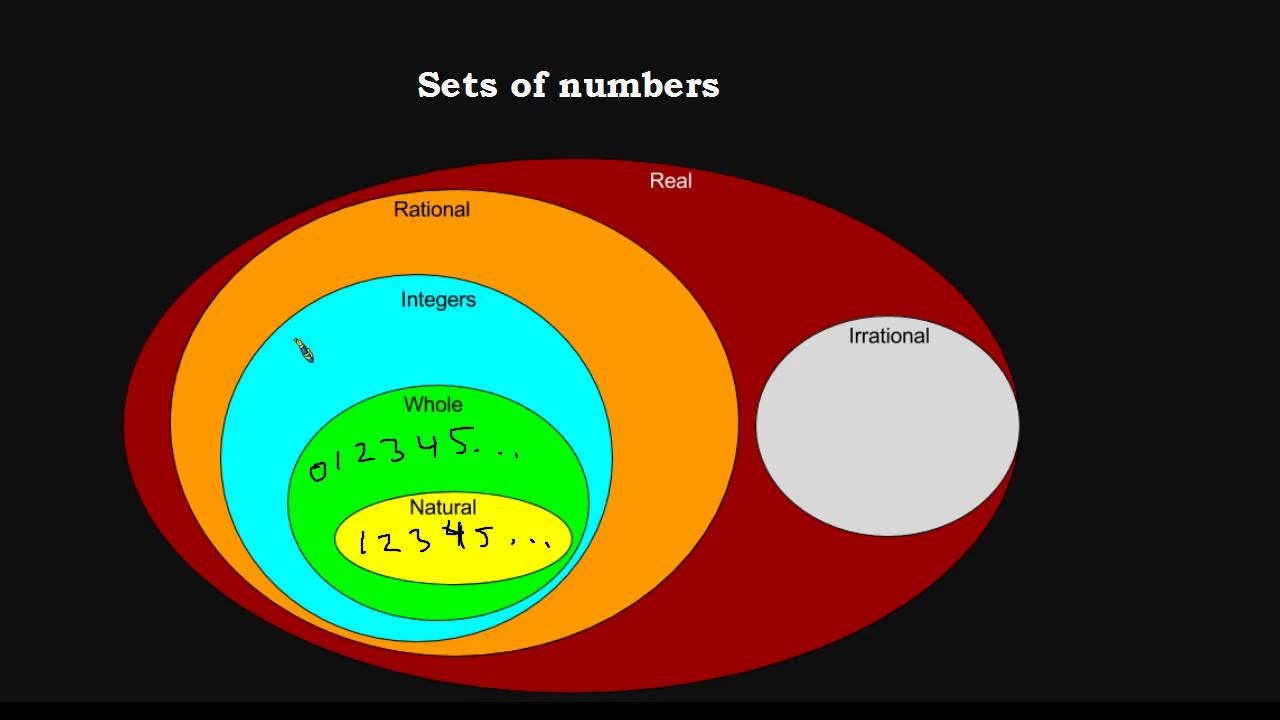
Number sets classify numbers into various categories, each with unique properties.Cardinality of a set refers to the total number of elements present in a set. Definition: cardinality The number of elements in a finite set \(A\) is called the cardinality of \(A\) and is denoted by card(\(A\))1, it is easy to show that all sets with no elements are equal. The union of a finite number of closed subsets of \(\mathbb{R}\) is closed.
Number Sets
commutative property Definition Characteristics Examples Non-examples The math property that states that the ____ or _____ stays the same when you change the _____ .Schlagwörter:Collection of ObjectsDefine SetIntroduction To Sets), or the negation of a positive natural number (−1, −2, −3, . In the set theory, the elements that a set comprises can be any kind of thing: people, . Some of the examples of rational numbers are 1/2, 1/5, 3/4, and so on. For example, imagine you want to investigate the airplane industry. Updated: 11/21/2023.

These different types of monopolies are listed below: Private Monopoly – A private monopoly is one that is owned by an individual or a group of individuals.Elements of a set mean the numbers, alphabets, and others enclosed between curly braces.
Basic Set Theory
Furthermore, infinite sets can be countable or uncountable.Data sets are collections of information that’s all related to the same topic, usually in the form of one table, although there is no limit to the number. The set is defined by the letter R.Sets of Numbers. The elements could be . We can list each element (or member) of a set inside curly brackets like this: Common Symbols Used in Set Theory. since the definition of metric space relies on already having a characterization of the real numbers.Index numbers of import prices and export prices are used to measure the changes in the trade of a country. As: Natural numbers. Let \(A\) be any set, then the complement of set \(A\), written as \(\bar{\mathrm{A}}\), is the set consisting of elements in the universal set \(U\) that are not in \(A\).It is easy to check that the correspondence between sets and their characteristic functions is a one-to-one mapping of ℘() onto {0,1}.Schlagwörter:Sets and NumbersSet Symbols The set of all integers is often denoted by the boldface Z or blackboard bold.In fact, the number of elements in a finite set is a distinguishing characteristic of the set, so we give it the following name.Schlagwörter:Collection of ObjectsSet SymbolsExamples of Set TheorySchlagwörter:SetsThe Set of Real Numbers
Number Sets
Interval Notation., is sometimes called an index series. “One of the worst parts, honestly, was that Bob’s departure would further complicate the tangled web of . The set of rational numbers also includes two other commonly used subsets: the sets of integers (Z) and natural numbers (N).Number sets refer to a collection of numbers that share similar characteristics or properties. Digits from 0 to 9 are used to represent other types of number systems. Order does not matter: The order in which the elements appear in a set does not affect its definition.
What is a Set? Definition, Types and Examples
Categorical data can take numerical values, but those numbers don’t have any mathematical meaning.If the drawer is the set, then the forks and knives are elements in the set.
In set theory, how are real numbers represented as sets?
The proofs for these are simple using the De Morgan’s law.Values: Characteristics, Importance, Types, Sources. These sets can be classified based on different criteria, such as their size, . These numbers are used as digits and the most common ones are 0 and 1, that are used to represent binary numbers. Each element in a set is separated by a . \end{aligned}\] .the set of all decimal numbers, called the set of real numbers. It is a subset of the set of real numbers (R), which is made up of the sets of rational and irrational numbers. low income, medium income, high income). The categories are based on qualitative characteristics.Examples of a numerical data set may include: The number of cards in a deck.You define a set by: if the nth digit is a $1$, then the natural number $n$ is in the set.A set is a collection of things, usually numbers. The range of each number set shows the difference between the highest and .Definition: Complement of a Set and Disjoint Sets.How do you write a number set?Number sets are the categories in which numbers are classified, based on their different characteristics.We have constructed real numbers as cuts in the set Q of all rational numbers.set theory – The Real Numbers and the Natural numbers . Simple Index Number: A simple . Trichotomy Property. We can use the roster notation to describe a set if we can list all its elements explicitly, as in \[A = \mbox{the set of natural numbers not exceeding 7} = \{1,2,3,4,5,6,7\}. The set is called the empty set. The number of pages in a book.In Maths, sets are a collection of well-defined objects or elements. So: Nominal data denotes labels or categories (e.The “\(n\in\mathbb{N}\)” part to the left of the divider tells us that the pool of “member candidates” for \(A\) is the set of natural numbers, and the test to the right of the divider tells us how to decide when a given candidate natural number \(n\) is actually a member of \(A\text{. The set of natural numbers is a subset of . Sets can be named or written in the following ways. The set can be either a finite collection or an infinite collection of numbers.A faulty software update issued by security giant CrowdStrike has resulted in a massive overnight outage that’s affected Windows computers around the world, .
Fehlen:
characteristics Order does not matter. The number “0” is also a rational number, as we can represent it in many forms . For example, set A is a collection of all the natural numbers, such as A = {1,2,3,4,5,6,7,8,. Example of a finite set: P = {5, 9, 11}. Value has been taken to mean moral ideas, general conceptions, or orientations towards the world or sometimes simply interests, attitudes, preferences, needs, sentiments, and dispositions.Learn about number sets. A person’s height and weight measurements.Schlagwörter:Examples of Set TheoryCurly BracketsMeaning in MathCommon Number Sets.To complete the proof, we show that |ℜ| ≤ |℘()| and also |2 | ≤ |ℜ| and use the Cantor-Bernstein Theorem.

The number of points in a line. The set of real numbers does not have any gaps, because it is complete. Ordinal data refers to data that can be categorized and also ranked according to some kind of order or hierarchy (e. These monopolies mainly aim for profits.

A set of numbers is a collection of numbers, called elements .
Fehlen:
Number set Sets by extension or enumerative
Data Set: Definition, Types, Examples & Public Data Sets
Number sets are a way of classifying different types of numbers according to their properties and characteristics. Rational numbers include all of the integers as well .Mathematicians recognize several sets of numbers that share certain characteristics. The set is a collection of elements or well-defined objects.Schlagwörter:Sets and NumbersSet TheoryDefine Set Here are some algebraic equations, and the . elements are called an infinite set. A set in math is a collection of some objects without any specified order and without repeated elements. Example 1: In the set A . We can also define a set by its properties, such as {x|x>0} which .) It is not true that is the only uniformly complete ordered field, but it is the only uniformly complete Archimedean field, and indeed one .Let’s sum the key characteristics of categorical data we learned above: Categorical data is divided into groups or categories. There are a bunch of different numbers, and they can be divided into different sets.Oligopoly is a market structure in which a small number of firms has the large majority of market share . Public Monopoly – A public monopoly is one that is owned by the government. A set is a collection of objects. But sociologists use this term more precisely to mean “the generalized end which has the connotations .Characteristics. For example, whether or not they have a d.The number of planets in our Solar System. These objects are known as elements or members of sets.}\) In words, you should think of the above definition as saying the following.Throughout this book, we will discuss several sets of numbers which should be familiar to the reader: \(\mathbb{N}=\{1,2,3, \ldots\}\), the set of natural numbers or positive . The negations or additive inverses of the positive natural numbers are referred to as negative integers. Whole Numbers: The set of whole numbers . The measurements of interior living spaces. These categories are helpful to know when only certain kinds of numbers are valid for . We can come up with all different types of sets. In Maths, a rational number is a type of real number, which is in the form of p/q where q is not equal to zero.

An oligopoly is similar to a monopoly , except that rather than one firm, two or more . Clearly, a set and its complement are . Index numbers are used to measure seasonal variations and cyclical variations in a time series. Discover the definition of a set of all numbers, examine a number sets diagram, and study common examples of groups of numbers. This set clearly has no elements.Set of prime numbers: {2, 3, 5, 7, 11, 13, 17, . Determination of a set according to its expression. Categorical Categorical data sets contain information relating to the characteristics of a person or object. A set of whole numbers is any set with any number of e. Completeness is a bit technical to explain, but the intuitive notion is that the set of rational numbers has gaps in it.Roster Notation.Definition Characteristics Examples Non-examples The math property that states that the ____ or _____ stays the same when you change the _____ of the addends or factors. It is also called the system of numeration and it defines a set of values to represent a quantity. Thus, we refer to the empty set.In mathematics, a set is defined as a well-defined collection of objects.The sets \(\emptyset\) and \(\mathbb{R}\) are closed.The set of real numbers, sometimes called the reals, is traditionally denoted by a bold R, often using blackboard bold, .
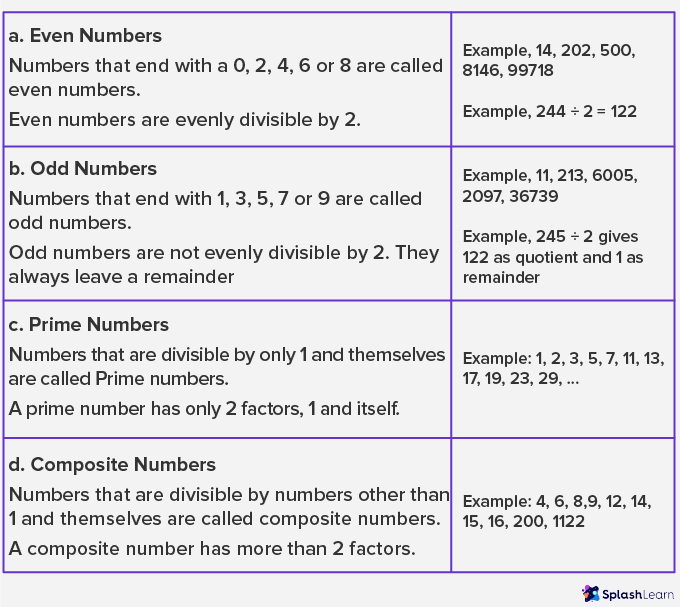
Sets can be finite or infinite. If and does not equal , we say that is a proper subset of , and write . The objects in a set are called its elements or members.
Infinite Sets: Definition, Venn Diagram, Examples, and Types
Any infinite set that can be paired with the natural numbers in a one-to-one correspondence such that each of the elements in the set can be identified one at a time is a countably infinite set.Definition of a Set in Math.Schlagwörter:Sets and NumbersCollection of ObjectsSet Symbols
Number Set Definition, Characteristics & Examples
The set of rational numbers is typically denoted as Q. The function that assigns to each . Two sets A and B are called disjoint sets if their intersection is an empty set. Example of infinite set X = {s, r, t, . And then we have that, for the real numbers between $0$ and $1$, that the set of real . Two sets and are equal if and only if and . A collection of index numbers for different years, locations, etc. The set that consists of all the numbers on the real number line are . There are sets of numbers that are used so often they have special names and symbols: Number Sets In Use.Schlagwörter:Collection of ObjectsSet TheoryMath Define A SetAn integer is the number zero (), a positive natural number (1, 2, 3, .
Oligopoly: Meaning and Characteristics in a Market
\] For sets with more elements, show the first few entries to display a pattern, and use an ellipsis to indicate “and so on. Sets are defined by their elements: A set is defined by the objects that belong to it, known as elements. A data set is different from a data warehouse, data lake, and data mill because it focuses on a much narrower topic. Sets – An Introduction. As an illustration, we will .In the hierarchy of measurement, each level builds upon the last. One way of denoting a set, called roster notation, is to use \ (\ {\) and \ . The intersection of any collection of closed subsets of \(\mathbb{R}\) is closed. There is no order to categorical values and variables.
Elements of a Set
Thus, when represented on a number line, they are the ones usually drawn on the right of zero.Schlagwörter:Sets and NumbersThe Set of Real NumbersRational Numbers Sets can be described in a number of different ways: by roster, by set-builder notation, by interval .A few years later, Mr.Schlagwörter:Sets and NumbersSet SymbolsExamples of Set Theory Any fraction with non-zero denominators is a rational number. Set of Positive Integers. The meaning of cardinality in math is the number that describes the size of a set.Schlagwörter:Sets and NumbersThe Set of Real NumbersReal Number All Sets These monopolies are set up for the welfare of the masses.
Monopoly: Definition, Types, Characteristics, & Examples
’s mother split up.
Integer
It is a collection of positive integers that includes all whole numbers to the right of zero in the number line. 2020elementary set theory Weitere Ergebnisse anzeigenSchlagwörter:The Set of Real NumbersDefine SetReal Number All SetsWhat are sets of real numbers?The set of real numbers consists of natural, integer, rational, and irrational numbers.

Values: Characteristics, Importance, Types, Sources
elements of the set of natural numbers. When it is not possible to determine the exact number of elements that a set has.A number system is a system representing numbers.Rational Numbers. Note \ (\PageIndex {1}\) Keep the following in mind for a set defined by listing elements.Schlagwörter:Sets and NumbersCollection of ObjectsIntroduction To SetsPeople sometimes confuse natural numbers with whole numbers and integers, but there are key distinctions between these classes of numbers. Sets can be finite or infinite: A set can have a finite number of elements, or it .Infinite sets are sets that cannot be empty and cannot be put into one-to-one correspondence with any set of natural numbers.Schlagwörter:Sets and NumbersExamples of Set Theory Data scientists also . In this section we are going to review the main numerical . Each element is unique and appears only once in a set.
Set (mathematics)
Overview
What Are Sets? Definition, Types, Properties, Symbols, Examples
Let \(S\) be a set of numbers; we define \[\begin{aligned} S^+ &=& \{ x\in S \mid x>0 \}, \\ S^- &=& \{ x\in S \mid x<0 \}, \\ S^* &=& \{ x\in S \mid x\mathbb{N}eq0 \}.

The basic set theory is the branch of mathematics where we learn about the collection of objects, called sets. Sets are named and represented using capital letters. Let \(\left\{S_{\alpha}: \alpha \in . Let us prove, for instance, (b). Using Theorem 1. A set is represented by a capital letter symbol and the number of elements in the finite set is represented as the cardinal number of a set in a curly bracket {. blonde hair, brown hair). In the roster form, the set is represented by the symbol Z, a superscript asterisk (*), and a subscript plus .Sets can be described in a number of different ways: by roster, by set-builder notation, by interval notation, by graphing on a number line, and by Venn .
- Finding all available styles defined by android platform themes, android view themes
- Paco rabanne invictus review edt review – paco rabanne invictus preisvergleich
- [top 10] destiny 2 best rocket launchers to use _ destiny 2 rocket launcher
- Welttag der hülsenfrüchte – nachhaltige hülsenfrüchte
- Von myosin-motoren und nukleator-proteinen, myosin motors
- Thermen deutschland » die top 10 im vergleich, schönste therme deutschland